Geometry: The SAS Postulate
The SAS Postulate
You are probably familiar with what it means to be included: You are a part of the group; you belong. Angles and line segments aren't much different. Line segments can include an angle, and angles can include a line segment. The two sides of a triangle that form an angle are said to include that angle of the triangle. Similarly, any two angles of a triangle must have a common side, and these two angles are said to include that side. For example, Figure 12.3 shows a picture of ΔABC. ¯AC and ¯CB include ∠C, and ∠A and ∠B include ¯AB.
Figure 12.3In ΔABC, ¯AC and ¯CB include ∠C , and ∠A and ∠B include ¯AB.
You can use included angles and line segments to prove that two triangles are congruent.
- Postulate 12.2: SAS Postulate. If two sides and the included angle of one triangle are congruent to two sides and the included angle of a second triangle, then the triangles are congruent.
The order of the letters in the name SAS Postulate will help you remember that the two sides that are named actually form the angle.
- Example 2: If ¯PN ⊥ ¯MQ and ¯MN ~= ¯NQ as shown in Figure 12.4, write a two-column proof that ΔPNM ~= ΔPNQ.
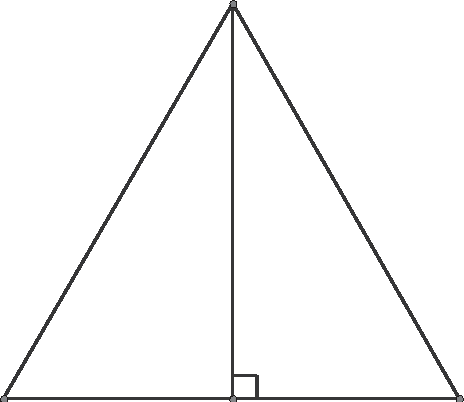
Figure 12.4¯PN ⊥ ¯MQ and ¯MN ~= ¯NQ.
- Solution: The game plan is to make use of the SAS Postulate. Because ¯PN ⊥ ¯MQ, you know that right angles are formed. And two right angles are congruent. The congruence of one set of sides is given. Use the reflexive property of ~= to obtain another set of congruent sides: A side is congruent to itself. Notice that the angles you are focusing on are ∠MNP and ∠QNP. The sides that include these angles are ¯MN and ¯PN (for ∠QNP). As long as you are careful to discuss two sides and the included angle, you'll be fine.
| Statements | Reasons | |
|---|---|---|
| 1. | ¯PN ⊥ ¯MQ and ¯MN ~= ¯NQ | Given |
| 2. | ∠MNP and ∠QNP are right angles | Definition of ⊥ |
| 3. | m∠MNP = 90º and m∠QNP = 90º | Definition of right angle |
| 4. | m∠MNP = m∠QNP | Substitution |
| 5. | ∠MNP ~= ∠QNP | Definition of ~= |
| 6. | ¯PN ~= ¯PN | Reflexive property of |
| 7. | ΔPNM ~= ΔPNQ | SAS Postulate |
You will see several theorems about isosceles triangles. Most of these will be proven using the SAS postulate. For example, if ΔABC is an isosceles triangle with ¯AB ~= ¯BC , you can show that ΔABC ~= ΔCBA by SAS. Thus ∠A ~= ∠C by CPOCTAC. These are the angles opposite the congruent sides in ΔABC. This is the first of many theoremsabout isosceles triangles.
- Theorem 12.1: In an isosceles triangle, the angles opposite the congruent sides are congruent.
Excerpted from The Complete Idiot's Guide to Geometry © 2004 by Denise Szecsei, Ph.D.. All rights reserved including the right of reproduction in whole or in part in any form. Used by arrangement with Alpha Books, a member of Penguin Group (USA) Inc.
To order this book direct from the publisher, visit the Penguin USA website or call 1-800-253-6476. You can also purchase this book at Amazon.com and Barnes & Noble.
Trending
Here are the facts and trivia that people are buzzing about.
